Question

For air travelers, one of the biggest complaints is of the waiting time between when the airplane taxis away from the terminal until the flight takes off. This waiting time is known to have a skewed-right distribution with a mean of 10 minutes and a standard deviation of 8 minutes. Suppose 100 flights have been randomly sampled. Describe the sampling distribution of the mean waiting time between when the airplane taxis away from the terminal until the flight takes off for these 100 flights.

Answer
Distribution is skewed-right with mean = 10 minutes and standard error = 0.8 minutes.
Distribution is skewed-right with mean = 10 minutes and standard error = 8 minutes.
Distribution is approximately normal with mean = 10 minutes and standard error = 0.8 minutes.
Distribution is approximately normal with mean = 10 minutes and standard error = 8 minutes.

Answer 1

Distribution is skewed-right with mean = 10 minutes and standard error = 0.8 minutes.

Explanation:

The problem states that

This waiting time is known to have a skewed-right distribution, so in the answer, the distribution is skewed right.

Mean of 10 minutes. Each alternative has this.

Here is the important part.

We have a sample of 100 flights. So we have to calculate the standard deviation of the sample.

A sample of length N of a distribution with standard deviation
`\sigma` has the following standard deviation.

`s = (\sigma)/(√(N))`

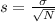
In this problem, we a have a sample of 100 flights with a standard deviation of 8 minutes. So

`s = (8)/(√(100)) = 0.8`

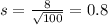
The correct answer is

Distribution is skewed-right with mean = 10 minutes and standard error = 0.8 minutes.