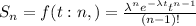Answer:
The distribution is
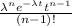
Solution:
As per the question:
Total no. of riders = n
Now, suppose the
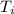 is the time between the departure of the rider i - 1 and i from the cable car.
is the time between the departure of the rider i - 1 and i from the cable car.
where
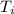 = independent exponential random variable whose rate is
= independent exponential random variable whose rate is

The general form is given by:
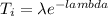
(a) Now, the time distribution of the last rider is given as the sum total of the time of each rider:
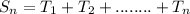
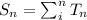
Now, the sum of the exponential random variable with
 with rate
with rate
 is given by:
is given by: