Answer:
600 tires/week OR dx/dt=-3/5 thousand tires/week
Step-by-step explanation:
where x is measured in units of a thousand and p is in dollars. $ p - \dfrac{1}{2}x^2 = 48 $ How fast is the weekly supply of Super Titan radial tires being introduced into the marketplace when x = 5, p = 60.5, and the price/tire is decreasing at the rate of $3/week?
p-1/2x^2=48
differentiating both sides with respect to time
d/dt(p-1/2x^2)=d/dt(48)
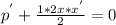
dp/dt+x dx/dt=0
-3=5dx/dt
dx/dt=-3/5
recall that x is measured in units of thousands
dx/dt=-0.6*1000
dx/dt=600 tires/week
OR dx/dt=-3/5 thousand tires/week
the 600 quantity supply of Super Titan radial tires decreases per each week