Answer:
There is a 69.15% probability that it weighs more than 0.8535 g.
Explanation:
Problems of normally distributed samples can be solved using the z-score formula.
In a set with mean
 and standard deviation
and standard deviation
 , the zscore of a measure X is given by
, the zscore of a measure X is given by

After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X. Subtracting 1 by the pvalue, we This p-value is the probability that the value of the measure is greater than X.
In this problem, we have that
The weights of a certain brand of candies are normally distributed with a mean weight of 0.8547 g, so
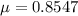 .
.
We have a sample of 463 candies, so we have to find the standard deviation of this sample to use in the place of
 in the Z score formula. We can do this by the following formula:
in the Z score formula. We can do this by the following formula:
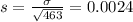
Find the probability that it weighs more than 0.8535
This is 1 subtracted by the pvalue of Z when
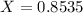
So
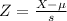
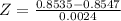
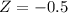
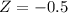 has a pvalue of 0.3085.
has a pvalue of 0.3085.
This means that there is a 1-0.3085 = 0.6915 = 69.15% probability that it weighs more than 0.8535 g.