Answer:
-7/25
Explanation:
 is in quadrant two given that
is in quadrant two given that
 is between 90 degrees and 180 degrees.
is between 90 degrees and 180 degrees.
This means cosine value there is negative and sine value is positive.
Let's use the Pythagorean Identity:
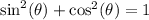 .
.
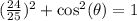
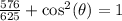
Subtract 576/625 on both sides:
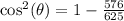

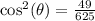
Take the square root of both sides:
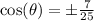
So recall that the cosine value here is negative due to the quadrant we are in.

Check:

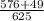
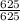

So we got the desired result since the right hand side of our Pythagorean Identity is 1.