Question

. (25 points) Suppose the probability of exposure to the flu during an epidemic is 0.6. A vaccine is 80% successful in preventing an inoculated person from acquiring the flu, if exposed to it. A person not inoculated faces a probability of 0.9 of aquiring the flu, if exposed to it. Define the probability of at least one will be flue for a randomly selected person.

Answer 1

The probability of at least one person will catch the flu is 0.5952

Explanation:

To find the probability of al least one catches the flu, we need to find the difference between 1 and the probability of not catching the flu.

The probability of catching the flu varies if the person is inoculated or not.

Let the events be:

E: the person was exposed

F: the person catches the flu

Hence, it is attached a tree diagram of the sample space. According to the diagram:

Inoculated person:

P(F')= 0.6×0.8 + 0.4×1 = 0.88 (We choose the second and the fourth way)

Not inoculated person:

P(F')= 0.6×0.1 + 0.4×1= 0.46 (We choose the second and the fourth way)

Let the events be:

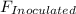`F_(Inoculated)`= the person is inoculated and catches the flu

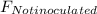`F_(Not inoculated)`=the person is not inoculated and catches the flu

Using complementary events, DeMorgan’s Laws, and independence we have:

P(
`F_(Inoculated)`∪
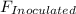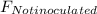`F_(Not inoculated)`)=1-P(
`F_(Inoculated)`∪
`F_(Not inoculated)`)'

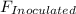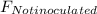=1 - P(
`F'_(Inoculated)`∩
`F'_(Not inoculated)`)

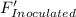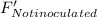=1 - P(
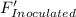`F'_(Inoculated)`)×P(
`F'_(Not inoculated)`)

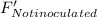=1 - (0.88)×(0.46)

=1 - 0.4048

=0.5952

The probability of at least one will catch the flu for a randomly selected person is 0.5952