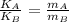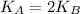Answer:
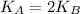
Step-by-step explanation:
As we know that initial height of both the balls are same
also the mass of the two balls is given as
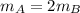
so here we can say by mechanical energy conservation
initial total mechanical energy = final total mechanical energy
since both balls are initially at rest so initial total kinetic energy of the balls will be zero
now final total kinetic energy = initial total potential energy
now we say

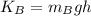
so we have