Answer:
C.I. = (2.297, 11.703)
Explanation:
The t-statistic for difference of mean is given by,

Here,
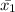 = 84
= 84
 = 7
= 7
s₁ = 4
n₁ = 12
s₂ = 6
n₂ = 18
Substituting all value in formula,
We get, t = -3.541 at 28 degree of freedom.
Using this formula, we get, t = 1.5342
Therefore, based on the data provided, the 99% confidence interval for the difference between the population means
 is: 2.297 <
is: 2.297 <
 < 11.703
< 11.703
which indicates that we are 99% confident that the true difference between population means is contained by the interval (2.297, 11.703)