Question

Paul has $30,000 to invest. His intent is to earn 15% interest on his investment. He can invest part of his money at 8% interest and part at 18% interest. How much does Paul eed to invest in each option to make a total 15% return on his $30,000?

A) 6% interest
B) 15% interest

Answer 1

Amount invested at 8% is $9000 and amount invested at 18% is $21000.

Explanation:

Let amount invested at 8% be x.

Let amount invested at 18% be y.

We get the 1st equation as:

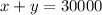`x+y=30000` ........(1)

We get the second equation as:

`0.08x+0.18(y)=0.15*30000`

=>
`0.08x+0.18y=4500`

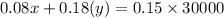
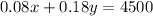
or getting rid of the decimal by multiplying by 100 on both sides.

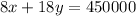`8x+18y=450000` ........(2)

Multiplying (1) by 8 and subtracting from (2) we get

`10y=210000`

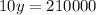
So, y = 21000

And
`x+21000=30000`

`x = 30000-21000`

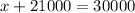
So, x = 9000

Therefore, amount invested at 8% is $9000 and amount invested at 18% is $21000.