Question

Carbon dioxide, which is recognized as the major contributor to global warming as a "greenhouse gas," is formed when fossil fuels are combusted, as in electrical power plants fueled by coal, oil, or natural gas. One potential way to reduce the amount of CO2 added to the atmosphere is to store it as a compressed gas in underground formations. Consider a 1000-megawatt coal-fired power plant that produces about 6×106 tons of CO2 per year. Part A Assuming ideal gas behavior, 1.00 atm, and 37 ∘C, calculate the volume of CO2 produced by this power plant.

Answer 1

The volume of CO₂ produced by this power plant is 3,466x10¹²L

Step-by-step explanation:

To obtain the volume of CO₂ produced you must use ideal gas law:

V = nRT/P

Where n are moles, R is gas constant (0,082atmL/molK), T is temperature and P is pressure (1,00atm)

The moles of CO₂ are:

6x10⁶ tons CO₂×
`(1x10^(6)g)/(1ton)` ×
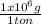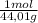`(1mol)/(44,01g)` = 1,363x10¹¹ moles of CO₂

Temperature in Kelvin is:

37°C + 273,15 = 310,15 K

Thus, the volume of CO₂ produced by this power plant is:

`V = 1,363x10^(11) mol*0,082atmL/molK*310,15K/1,00atm`

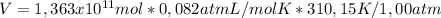
V = 3,466x10¹²L

I hope it helps!

Answer 2

Step-by-step explanation:

Let us assume that pressure is 1 atm and temperature is
`37^(o)C`.

Now, according to the ideal gas PV = nRT. Hence, number of moles will be calculated as follows.

n =
`\frac{mass}{\text{molar mass}}`

=
`(6,350,293,180,000)/(44.01)`

=
`1.443 * 10^(11)` mol

Also, convert temperature into kelvin as follows.

(273 + 37) K

= 310 K

Hence, calculate the volume as follows.

PV = nRT

V =
`(1.443 * 10^(11) * 0.0821 Latm/mol K * 310 K)/(1 atm)`

=
`3.67 * 10^(12)` L

Thus, we can conclude that volume of carbon dioxide produced by this power plant is
`3.67 * 10^(12)` L.