Question

A bullet is fired horizontally from a handgun at a target 100.0 m away. If the initial speed of the bullet as it leaves the gun is 300.0 m/s, how far vertically will the bullet have dropped by the time it hits the target? Ignore the effects of air resistance.

(A) 3.1 m
(B) 1.2 m
(C) 1.1 m
(D) 0

Answer 1

The distance is 0.53 m.

Step-by-step explanation:

Given that,

Target distance = 100.0 m

Speed of bullet = 300 m/s

We need to calculate the total time

Using formula of time

`t=(d)/(v)`

Put the value into the formula

`t=(100.0)/(300)`

`t=0.33\ sec`

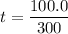
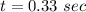
Now, consider vertical motion of bullet.

Initial velocity of bullet in vertical direction = 0 m/s

We need to calculate the vertically distance

Using equation of motion

`s=ut+(1)/(2)gt^2`

Put the value in the equation

`s=0+(1)/(2)*9.8*(0.33)^2`

`s=0.53\ m`

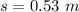
Hence, The distance is 0.53 m.