Question

Terry has a credit card that uses the average daily balance method for the first 18 days of one of his billing cycles, his balance was $350, and for the last 12 days of the billing cycle, his balance was $520. if his credit cards APR is 14% which of these expressions could be used to calculate the amount Terry was charged in interest for the billing cycle?

Answer 1

{0.14/365 • 30}{18 • $350 + 12 • $520/ 30}

Explanation:

Answer 2

Amount terry was charged in interest for the billing cycle is $ 4.9 approximately.

SOLUTION:

Given, Terry has a credit card that uses the average daily balance method for the first 18 days of one of his billing cycles, his balance was $350, and for the last 12 days of the billing cycle, his balance was $520.

His credit cards APR is 14%

Using the average daily balance method, the amount to be used in calculating Theresa's interest is given by:

`\frac{\text { sum of product of number of days in bulling cycle and balance. }}{\text { moter }}=(18 * 350+12 * 520)/(18+12)\\\\=(12540)/(30)=418`

Therefore, the interest charge on Theresa for the biling cycle is given by:

`\frac{\text {amount } * A P R}{12 \text { months }}=(418 * 0.14)/(12)=4.877`

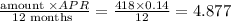
hence, amount terry was charged in interest for the billing cycle is $ 4.9 approximately.