Question

In Texas, 30% of parolees from prison return to prison within 3 years. Suppose 15 prisoners are released from a Texas prison on parole. Assume that whether or not one prisoner returns to prison is independent of whether any of the others return to prison. Let the random variable X be the number of parolees out of 15 that return to prison within 3 years. What are the values of the parameters for the binomial random variable X?

n =

p =

Answer 1

Answer: n= 15

p= 0.30

Explanation:

Binomial distribution :

Let x be a binomial variable.

The probability of getting success in x trials is given by :-

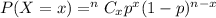`P(X=x)=^nC_xp^x(1-p)^(n-x)`, where n is the total number of trials and p is the probability of getting success in each trail.

Given : The proportion of parolees from prison return to prison within 3 years= 0.30

We assume that whether or not one prisoner returns to prison is independent of whether any of the others return to prison.

⇒ p =0.30

Let the random variable X be the number of parolees out of 15 that return to prison within 3 years.

⇒ n= 15