Question

A statistics professor plans classes so carefully that the lengths of her classes are uniformly distributed between 45.0 and 55.0 minutes.

A) Find the probability that a given class period runs between 51.5 and 51.75 minutes.
B) Find the probability of selecting a class that runs between 51.5 and 51.75 minutes.
(Round to three decimal places as needed.)

Answer 1

0.025

Explanation:

Hello!

Given that the classes are uniformly distributed between 45.0 and 55.0 minutes, the propability distribution will be:

`P(x)=(1)/(55-45) = (1)/(10)`

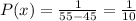
Now, we are looking for a probability P(51.5<x<51.75) which can be computed as:

`P(51.5<x<51.75) = \int\limits^(51.75)_(51.5) {P(x)} \, dx =(1)/(10)\int\limits^(51.75)_(51.5) {} \ dx\\\\P(51.5<x<51.75) = (51.75-51.5)/(10)=(0.25)/(10)`

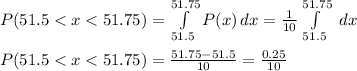
Therefore:

P(51.5<x<51.75) = 0.025

or

P(51.5<x<51.75) = 2.5%

I strongly believe that the answer for both questions have the same answer, I believe this because there is no additional info for a given class or selecting a class. I think both probabilities are the same.