Answer : The figure shows a line tangent to the circle is, 3rd figure
Step-by-step explanation :
As we know that, a tangent is a line segment to a circle which intersect the circle at exactly one point and that point is a tangency point.
And the radius from the center of the circle to the point of tangent is always perpendicular to the tangent line.
First we have to prove that the triangle formed by tangent is a right angled triangle.
Using Pythagoras theorem for 1st figure:
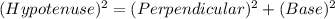
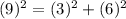
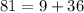
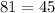
Using Pythagoras theorem for 2nd figure:
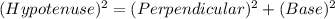
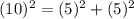
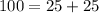
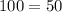
Using Pythagoras theorem for 3rd figure:
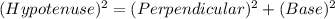

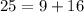
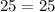
From this we conclude that, 3rd figure is a right angled triangle that means the radius from the center of the circle to the point of tangent is always perpendicular to the tangent line.
Hence, the figure shows a line tangent to the circle is, 3rd figure