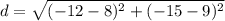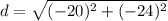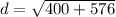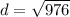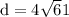Answer:
The distance of AB is
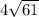
Solution:
In the question it is given that the midpoint M is (-2,-3) and point A is (8,9). To find the distance between A and B we have to find the point of B. Let the point of B be

We know,
Mid point for
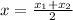
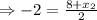
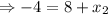
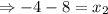
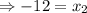
Midpoint



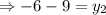
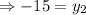
Therefore, the point of B is (-12,-15)
Using the distance formula between two point A and B which is given by
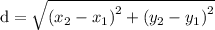
where d is the distance