Question

Benzyl alcohel can dissociate into benzaldehyde and hydrogen CeHsCH2OH(g) CeHsCHO(g)+Hz(g) At 250 °C, the equilibrium constant in terms of pressures, Ke for this dissociation reaction is 0.558 (a) If 11.56 g of benzyl alcohol is placed in a 19.85 L vessel and heated to 250 °C, what is the partial pressure of benzaldehyde when equilibrium is attained? stm (b) What fraction of benzyl alcohol is dissociated at equilibrium? Submit Answer 2 question attempts remaining

Answer 1

a) Partial pressure of benzyl alcohol is 0,23 atm

b) Fraction of benzyl alcohol dissociated at equilibrium is 0,99

Step-by-step explanation:

The dissociation of benzyl alcohol is:

C₆H₅CH₂OH(g) ⇄ C₆H₅CHO(g) + H₂(g)

With ke = [C₆H₅CHO] [H₂] / [C₆H₅CH₂OH]

The molarity of benzyl alcohol is:

11,56g×
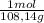`(1mol)/(108,14 g)` = 0,1069 moles of benzyl alcohol/19,85L = 5,385x10⁻³M

The concentrations in equilibrium after the addition of benzyl alcohol are:

[C₆H₅CH₂OH] = 5,385x10⁻³M - x

[C₆H₅CHO] = x

[H₂] = x

Where x are the moles produced of both benzaldehyde and hydrogen chemicals.

Replacing:

0,558 = [C₆H₅CHO] [H₂] / [C₆H₅CH₂OH]

0,558 = [x] [x] / [5,385x10⁻³-x]

x² + 0,558x - 3x10⁻³

Solving:

x = -0,5633No chemical sense. There are not negative concentrations

x = 0,00533 Real answer.

a) Molarity of benzaldehyde is 0,00533

By ideal gas law:

P = M RT

Where P is pressure

M is molarity (0,00533M)

R is gas constant (0,082atmL/molK)

T is temperature (250+273,15 = 523,15K)

Replacing, partial pressure of benzyl alcohol is P = 0,23 atm

b) The benzyl alcohol dissociated is 0,00533M. The initial molarity of benzyl alcohol is 5,385x10⁻³M. Thus, fraction of benzyl alcohol dissociated at equilibrium is 0,00533/5,385x10⁻³ = 0,99

I hope it helps!