Answer: The value of
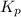 for the reaction is 6.32 and concentrations of
for the reaction is 6.32 and concentrations of
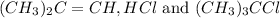 is 0.094 M, 0.094 M and 0.106 M respectively.
is 0.094 M, 0.094 M and 0.106 M respectively.
Step-by-step explanation:
Relation of
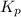 with
with
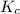 is given by the formula:
is given by the formula:
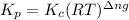
where,
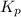 = equilibrium constant in terms of partial pressure = 3.45
= equilibrium constant in terms of partial pressure = 3.45
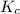 = equilibrium constant in terms of concentration = ?
= equilibrium constant in terms of concentration = ?
R = Gas constant =

T = temperature = 500 K
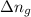 = change in number of moles of gas particles =
= change in number of moles of gas particles =
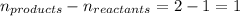
Putting values in above equation, we get:
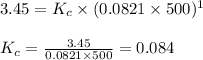
The equation used to calculate concentration of a solution is:

Initial moles of
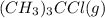 = 1.00 mol
= 1.00 mol
Volume of the flask = 5.00 L
So,
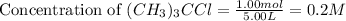
For the given chemical reaction:
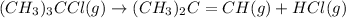
Initial: 0.2 - -
At Eqllm: 0.2 - x x x
The expression of
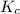 for above reaction follows:
for above reaction follows:
![K_c=([(CH_3)_2C=CH]* [HCl])/([(CH_3)_3CCl])](https://img.qammunity.org/2020/formulas/chemistry/college/jiehpvgoiep48m6i8wsruip3pyqgf50ahr.png)
Putting values in above equation, we get:
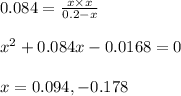
Negative value of 'x' is neglected because initial concentration cannot be more than the given concentration
Calculating the concentration of reactants and products:
![[(CH_3)_2C=CH]=x=0.094M](https://img.qammunity.org/2020/formulas/chemistry/college/7nviyik7afq0g6wkylir1tk5t4q8tow12l.png)
![[HCl]=x=0.094M](https://img.qammunity.org/2020/formulas/chemistry/college/53k6ducq0tlcho7gudfy2kqype3gx10i3g.png)
![[(CH_3)_3CCl]=(0.2-x)=(0.2-0.094)=0.106M](https://img.qammunity.org/2020/formulas/chemistry/college/llr7c7msq7q27927c7a74c3d888jtw0ju9.png)
Hence, the value of
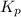 for the reaction is 6.32 and concentrations of
for the reaction is 6.32 and concentrations of
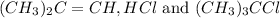 is 0.094 M, 0.094 M and 0.106 M respectively.
is 0.094 M, 0.094 M and 0.106 M respectively.