Answer:
Andy went as furthest as possible with a gallon of gasoline
Explanation:
Let
m ----> the distance in miles
g ----> the number of gallons of gas
step 1
Kelley's Mileage
Find the slope of the linear function of Kelley's Mileage
we have the points
(0,0) and (20,50)
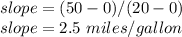
the linear equation is
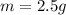
step 2
Andy's Mileage
Find the slope of the linear function of Andy's Mileage
we have the points
(15,78.75) and (30,157.5)
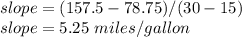
the linear equation is
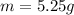
step 3
Rick's Mileage
we have
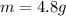
therefore
the slope is

step 4
Compare the slopes
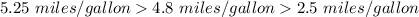
therefore
Andy went as furthest as possible with a gallon of gasoline