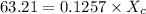Question

A 120 V, 60 Hz power source is connected in series across an 800 Ω resistance and an unknown capacitance in series. The voltage drop across the resistor is 102 V. Find (a) the voltage drop across the capacitor; and (b) the reactance of the capacitor.

Answer 1

(a) 63.21 volt

(b) 495.76 ohm

Step-by-step explanation:

We have given Voltage V = 120 Volt

Frequency f = 60 Hz

Resistance R = 800 ohm

Voltage drop across resistor
`V_R=102volt`

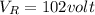
(A) We know that resultant voltage for series circuit is given by
`V=√(V_R^2+V_C^2)`

`120=√(102^2+V_C^2)`

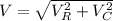
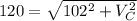
Squaring both side
`14400=10404+V_C^2`

`V_C=63.2139Volt`

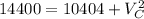
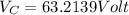
(b) Current through the circuit
`I=(V_R)/(R)=(102)/(800)=0.1257A`

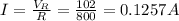
As
`V=IX_C`

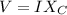
So
`63.21=0.1257* X_c`

`X_c=495.76ohm`

Answer 2

a)Vc= 63.21 V

b)Xc= 495.756 Ω

Step-by-step explanation:

Assume that voltage across the resistance is Vr and capacitor Vc

So

`V^2=V^2c+V^2r`

Now by putting the values

`120^2=V^2c+102^2`

Vc= 63.21 V

We know that for resistance

Vr= I R

102 = I x 800

I=0.1275 A

Let reactance of capacitor is Xc

So

Vc= Xc . I

63.21 = 0.1275 x Xc

Xc= 495.756 Ω