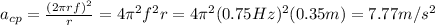Answer:
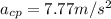
Step-by-step explanation:
The equation for centripetal acceleration is
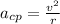 .
.
We know the wheel turns at 45 rpm, which means 0.75 revolutions per second (dividing by 60), so our frequency is f=0.75Hz, which is the inverse of the period T.
Our velocity is the relation between the distance traveled and the time taken, so is the relation between the circumference
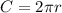 and the period T, then we have:
and the period T, then we have:
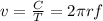
Putting all together: