Answer with Step-by-step explanation:
Let
A=Students are female
B=Students major in civil engineering
The probability that a students are female=P(A)=
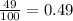
The probability that a student major in civil engineering=P(B)=
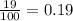
The probability that students both are female=
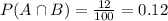
a.We have to find the probability that a random selected female student major in civil engineering.
We have to find
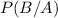
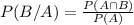
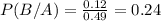
Hence, the probability that a randomly selected female student majors in civil engineering=0.24
b.We have to find the probability that a random selected civil engineering major is female.
We have to find
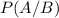
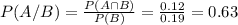
Hence, the probability that a randomly selected civil engineering major is female=0.63