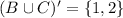Question

Lab Assinent 1 Page 2 of 2 62:261, Fall 2019 are subsets of the 4. Let A = {2,3,4,7), B = {4,6,7), C = (3,5,7,8,9), and note that A, B, and universe of discourse U = REZISIS 9} (a) (1 mark) Calculate AUB. (b) [1 mark] Find An B. (c) (1 mark) Find C (d) [1 mark) Calculate C-B. (e) (2 marks) Find (BU CY'.

Answer 1

a) AUB = {2,3,4,6,7}

b)
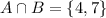
`A\cap B=\{4,7\}`

c)
`C'=\{1,2,4,6\}`

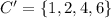
d)
`C-B=\{3,5,8,9\}`

e)
`(B\cup C)'=\{1,2\}`

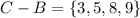
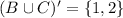
Explanation:

Consider the provided set.

A = {2,3,4,7}, B = {4,6,7}, C = {3,5,7,8,9}

Where A, B, and universe of discourse
`U=\1\leq x\leq 9\`

So
`U=\{1,2,3,4,5,6,7,8,9\}`

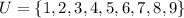
Part (A) Calculate AUB.

AUB represents the elements which are in A or in B or in both.

Therefore, AUB = {2,3,4,6,7}

Part (B) Find A n B

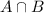`A\cap B` represent the elements which are in both the set A and B.

Therefore,
`A\cap B=\{4,7\}`

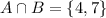
Part (C) Find C'

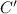`C'` represent the elements that are not in C.

So the set C' contains all the element of set U except 3,5,7,8,9

Therefore,
`C'=\{1,2,4,6\}`

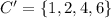
Part (D) Find C-B

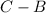`C-B` represent the elements of C which are not the elements of B.

So the set C-B contains all the element of set C except 7

Therefore,
`C-B=\{3,5,8,9\}`

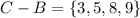
Part (E) Find (BUC)'

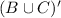`(B\cup C)'` represent all the elements of U which are not in B or in C or in both.

`B\cup C=\{3,4,5,6,7,8,9\}`

`(B\cup C)'=U-B\cup C=\{1,2\}`

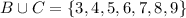
Therefore,
`(B\cup C)'=\{1,2\}`