Question

An angle in standard position measures −5π3 radians. In which quadrant does the terminal side of this angle lie?

Answer 1

Quadrant IV

Explanation:

Answer 2

The terminal side of this angle lies in the first quadrant.

Solution:

Given, an angle in standard position measures −5π/3 radians.

We need to find in which quadrant does the terminal side of this angle lie.

First let us convert -5π/3 radians into degrees.

`\text { Now, } (-5 \pi)/(3) \text { radians }=(-5 \pi)/(3) * (180)/(\pi) \text { degrees }`

`=(-5)/(3) * 180 \text { degrees }`

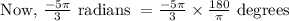
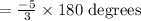
= -5 x 60 degrees = -300 degrees

Here, -300 represents that terminal side is rotating in anti clock wise direction. So now to find the positive angle.

Positive angle = 360 – 300 = 60 degrees

We know that, 0 degrees < 60 degrees < 90 degrees

Hence, the terminal side of this angle lies in the first quadrant.