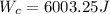Answer
Mass m = 78 kg
Vertical height in each stage h = 11 m
(a).
Initial speed u = 0
Final speed v = 1.1 m / s

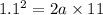
a = 0.055 m/s²
Work done
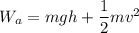
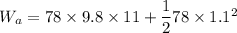
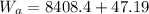
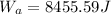
(b).Work done
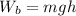
W_b = 78× 9.8× 11
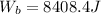
c)
Work done
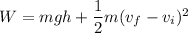
Where V = final speed
= 0
v = 1.1 m / s
for deceleration a = -0.055 m/s²
now,

W_c = 545.75 × 11