ANSWER:
If
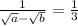 and
and
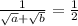 then the difference of a and b is 6
then the difference of a and b is 6
SOLUTION:
Given,
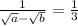 →
→
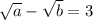 ----- (1)
----- (1)
And
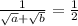 →
→
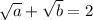 --- (2)
--- (2)
We have to find difference of a and b.
Now, add (1) and (2)
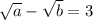
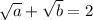
Adding above two equations, we get,
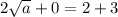
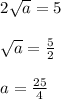
substitute
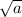 value in (2)
value in (2)
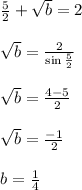
Now, difference of a and b is a – b =
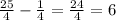
Hence, the difference of a and b is 6.