Question

An analyst will recommend a combination of 3 industrial stocks, 2 transportation stocks, and 2 utility stocks. If the analyst can choose from 5 industrial stocks, 4 transportation stocks, and 3 utility stocks, how many different combinations of 7 stocks are possible?

Answer 1

Answer:180

Explanation:

Given

There are 5 industrial stocks, 4 transportation stocks ,3 utility stocks

and analyst has to take 3 industrial stocks , 2 transportation stocks and 2 utility stocks out of above

No of ways of choosing 3 industrial stock out of 5 is
`^5C_3=10`

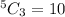
No of ways of choosing 2 transportation stock out of 4 is
`^4C_2=6`

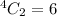
No of ways of choosing 2 utility stock out of 3 is
`^3C_2=3`

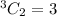
Thus total no of ways
`=^5C_3* ^4C_2* ^3C_2=10* 6* 3=180`

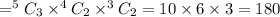
thus 180 combinations are possible