Question

A pilot flies in a straight path for 2 hours. He then makes a course correction, heading 15 degrees to the right of his original course and flies 1 hour in the new direction. If he maintains a constant speed of 575 miles per hour, how far is he from his starting position?

Answer 1

Answer:1711.8 miles

Step-by-step explanation:

Given

Pilot flies for 2 hr in straight line with speed of 575 mph

and deviates 15 right from its path

Considering Plane flies towards east

Position vector of pilot after 2 hr

`\vec{r_1}=575* 2\hat{i}`

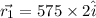
After 1 hr

`\vec{r_(21)}=575* 1\left ( cos15\hat{i}-sin(15)\hat{j}\right )`

`\vec{r_2}=\vec{r_(21)}+\vec{r_(1)}`

`\vec{r_2}=575* 1\left ( cos15\hat{i}-sin(15)\hat{j}\right )+575* 2\hat{i}`

`\vec{r_2}=1705.4\hat{i}-148.82\hat{j}`

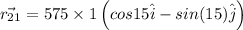
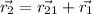
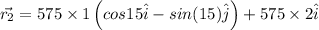
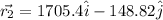
Distance from original position is given by magnitude of
`|r_2|`

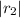
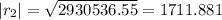`|r_2|=√(2930536.55)=1711.881` miles