Question

A common radio wavelength observed coming from astronomical objects is 21 cm. What temperature is associated with this radiation?

Answer 1

The temperature associated with this radiation is 0.014K.

Step-by-step explanation:

If we assume that the astronomical object behaves as a black body, the relation between its wavelength and temperature is given by Wien's displacement law.

`\lambda_(max)=(b)/(T)`

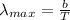
where,

λmax is the wavelength at the peak of emission

b is Wien's displacement constant (2.89×10⁻³ m⋅K)

T is the absolute temperature

For a wavelength of 21 cm,

`T=(b)/(\lambda _(max) ) = (2.89 * 10^(-3) m.K  )/(0.21m) =0.014K`