Question

Two people, one with mass m1 and the other with mass m2, stand on a stationary sled with mass M on a frozen lake. Assume that the ice is frictionless. a) The first person (with mass m1) jumps off the sled with a velocity −sxˆ relative to the (stationary) sled. What is his velocity? Use conservation of momentum to find the velocity of the sled (which is still carrying the second person). b) Now the second person jumps with the same velocity −sxˆ relative to the sled, which is now moving with the velocity you found in part a). What is her velocity? What is the new velocity of the sled? c) What is the change in the total kinetic energy of the two people and the sled

Answer 1

Part a)

Velocity of sled

`v = (m_1 s)/(m_1 + m_2 + M)`

velocity of first man who jump off

`v_1 = -((m_2 + M) s)/(m_1 + m_2 + M)`

Part b)

Velocity of sled

`v_f = ((m_1 s)/(m_1 + m_2 + M)) + ((m_2)/(m_2 + M))s`

Also the speed of second person is given as

`v_2 = ((m_1 s)/(m_1 + m_2 + M)) - (Ms)/(m_2 + M)`

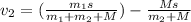
Part c)

change in kinetic energy of sled + two people is given as

`KE = (1)/(2)Mv_f^2 + (1)/(2)m_1v_1^2 + (1)/(2)m_2v_2^2`

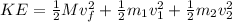
Step-by-step explanation:

As we know that here we we consider both people + sled as a system then there is no external force on it

So here we can use momentum conservation

since both people + sled is at rest initially so initial total momentum is zero

now when first people will jump with relative velocity "s" then let say the sled + other people will move off with speed v

so by momentum conservation we have

`0 = m_1(v - s) + (m_2 + M)v`

`v = (m_1 s)/(m_1 + m_2 + M)`

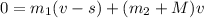
so velocity of the sled + other person is

`v = (m_1 s)/(m_1 + m_2 + M)`

velocity of first man who jump off

`v_1 = (m_1 s)/(m_1 + m_2 + M) - s`

`v_1 = -((m_2 + M) s)/(m_1 + m_2 + M)`

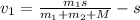
Part b)

now when other man also jump off with same relative velocity

so let say the sled is now moving with speed vf

so by momentum conservation we have

`(m_2 + M)((m_1 s)/(m_1 + m_2 + M)) = m_2(v_f - s) + Mv_f`

`(m_2 + M)((m_1 s)/(m_1 + m_2 + M)) + m_2s = (m_2 + M)v_f`

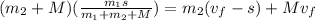
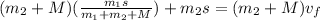
Now we have

`v_f = ((m_1 s)/(m_1 + m_2 + M)) + ((m_2)/(m_2 + M))s`

Also the speed of second person is given as

`v_2 = ((m_1 s)/(m_1 + m_2 + M)) + ((m_2)/(m_2 + M))s - s`

`v_2 = ((m_1 s)/(m_1 + m_2 + M)) - (Ms)/(m_2 + M)`

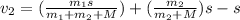
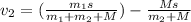
Part c)

change in kinetic energy of sled + two people is given as

`KE = (1)/(2)Mv_f^2 + (1)/(2)m_1v_1^2 + (1)/(2)m_2v_2^2`

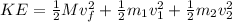
here we know all values of speed as we found it in part a) and part b)