Question

(1 point) A pilot flies in a straight path for 1 hour and 30 min. She then makes a course correction, heading 10 degrees to the right of her original course, and flies 2 hours in the new direction. If she maintains a constant speed of 635 miles per hour, how far is she from her starting position?

Answer 1

The pilot is 2214.22 miles from her starting position

Step-by-step explanation:

Since the pilot is traveling at a constant speed of 635 mph, the total distance traveled can be easily found as follows:

`1stleg: d_(1) = 1.5*635 = 952.5 mi\\2ndleg: d_(2) = 2*635 = 1270 mi`

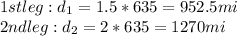
There was a 10 degrees deviation, so the angle between the trajectory of both legs is 170 degrees.

The distance we need to find is that from the start of the first leg to the end of the second leg, those three distances form a triangle and since the side we're interested in is opposite to the 170 degrees angle, we can determine its length by the law of cosines:

`d_(3)^(2) =d_(1)^(2) + d_(2)^(2) -2*d_(1)*d_(2)*cos(170)\\d_(3)^(2) =952.5^(2) + 1270^(2) -2*952.5*1270*cos(170)\\d_(3)=2214.22mi`

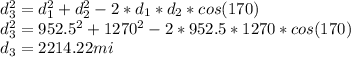
The pilot is 2214.22 miles from her starting position