Question

Find the odds for and the odds against the event rolling a fair die and getting a 6,2, or 1

Answer 1

1/6

Explanation:

Because in a dice it has 6 sides so it would be 1/6 for all of them

Answer 2

Odds for and odd against the given event of rolling a fair die is
`(1)/(2)` and
`(1)/(2)` respectively.

Solution:

Need to find the odds for and odd against the event of rolling a fair die and getting 6, 2, 1

Number of possible outcomes of rolling a fair die = 6

Number of favorable outcome that is getting 6,2 or 1 = 3

Number of non favorable outcomes that is getting 5, 4 or 3 = 3

`\text { Odd for getting a } 6,2, \text { or } 1=\frac{\text { Number of favorable outcome }}{\text { Number of possible outcomes }}`

`= (3)/(6) = (1)/(2)`

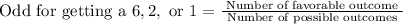
Odd against getting a 6, 2 or 1 means odds for getting 5, 4 or 3

`\text { So, odd against getting a } 6,2, \text { or } 1=\frac{\text { Number of non favorable outcomes }}{\text { Number of possible outcomes }}`

`= (3)/(6) = (1)/(2)`

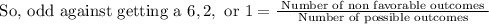
Hence odds for and odd against the given event of rolling a fair die is
`(1)/(2)` and
`(1)/(2)` respectively.