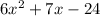Question

The domain for f(x) and g(x) is the set of all real numbers. Let f(x) = 2x-3 and g(x) = 3x +8 Find f(x)g(x). Please show work

Answer 1

The value of f(x)g(x) is
`6 x^(2)+7 x-24`

Solution:

The domain for f(x) and g(x) is the set of all real numbers

From question give that

f(x) = 2x – 3 ----- (1)

g(x) = 3x + 8 ------- (2)

We need to evaluate f(x)g(x), so we need to multiply eqn 1 and eqn 2

f(x)g(x) = (2x – 3)( 3x + 8) [Using equation (1) and (2)]

Open the bracket and multiply the terms in above equation,

`\begin{array}{l}{\mathrm{f}(\mathrm{x}) \mathrm{g}(\mathrm{x})=6 \mathrm{x}^(2)+16 \mathrm{x}-9 \mathrm{x}-24} \\ {\mathrm{f}(\mathrm{x}) \mathrm{g}(\mathrm{x})=6 \mathrm{x}^(2)+7 \mathrm{x}-24}\end{array}`

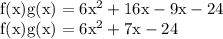
Hence the value of f(x)g(x) is
`6 x^(2)+7 x-24`