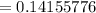Answer: 0.14155776
Explanation:
Given : The proportion of workers employed by the university belong to the workers' union=0.96
Let x be a binomial variable that represents that worker belongs to the workers' union.
Sample size : n= 4

[Binomial probability formula : P(X=x)=^nC_x(p)^x(1-p)^{n-x}]
Hence, the probability that exactly 3 of the workers interviewed are union members