Question

Can anyone help me with these two problems?

Answer 1

Show

`(\sin ^2 x + \sin x \cos x)/(\cos x - 2 \cos^3 x) = (\tan x)/(\sin x - \cos x)`

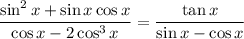
I don't like this computer aided instruction holding my hand. I'll ignore that at first so I can compare my solution to the one being taught.

We can see how a tan factors out; let's see what's left.

`(\sin ^2 x + \sin x \cos x)/(\cos x - 2 \cos^3 x)`

`=(\sin x)/(\cos x) \cdot ( \sin x + \cos x)/(1 - 2 \cos^2 x)`

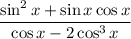
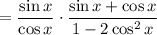
We need to factor out a sin x + cos x in the denominator so let's change one of the squared cosines to squared sine.

`= \tan x ( \sin x + \cos x)/(1 - \cos^2 x - (1 - \sin ^2 x))`

`= \tan x ( \sin x + \cos x)/(\sin^2 x - \cos^2 x)`

`= \tan x ( \sin x + \cos x)/((\sin x + \cos x)(\sin x - \cos x))`

`=(\tan x)/(\sin x - \cos x) \quad\checkmark`

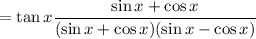
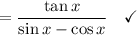
OK, now I can look at what they did.

First step ok, the box is 2 cos^2 x

Second step they changed the 1, box same as before: 2 cos^2 x

Third box matches our proof, cos^2 x

Fourth box our last box didn't change: cos^2 x

Fifth box: sin x + cos x