Question

A hot air balloon in flight begins to ascend at a steady rate of 120 feet per minute. After 1.5 minutes, the balloon is at an altitude of 2150 feet. After 3 minutes, it is at an altitude of 2330 feet. Use an equation in point-slope form to determine whether the balloon will teach an altitude of 2500 feet in 4 minutes.

Answer 1

The balloon will not reach 2,500 feet after 4 minutes.

Explanation:

The equation of the line in the point-slope form is

`y-y_1=m(x-x_1)`

A hot air balloon in flight begins to ascend at a steady rate of 120 feet per minute, then the slope is

`m=120`

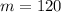
After
`x_1=1.5` minutes, the balloon is at an altitude of
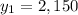`y_1=2,150` feet. This means, the line passes through the point (1.5, 2,150).

Substitute the slope and point coordinates into the equation:

`y-2,150=120(x-1.5)`

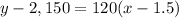
We know that after 3 minutes, the balloon is at an altitude of 2,330 feet. Check this:

`2,330-2,150=180\\ \\120(3-1.5)=120\cdot 1.5=180\\ \\2,330-2,150=120(3-1.5)`

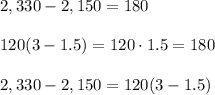
Now, find the height after 4 minutes:

`y-2,150=120(4-1.5)\\ \\y=120\cdot 2.5+2,150=300+2,150=2,450`

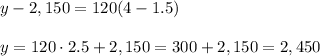
Thus, the balloon will reach only 2,450 feet after 4 minutes.