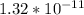Question

Ask Your Teacher Scientific work is currently underway to determine whether weak oscillating magnetic fields can affect human health. For example, one study found that drivers of trains had a higher incidence of blood cancer than other railway workers, possibly due to long exposure to mechanical devices in the train engine cab. Consider a magnetic field of magnitude 0.00100 T, oscillating sinusoidally at 69.5 Hz. If the diameter of a red blood cell is 6.20 µm, determine the maximum emf that can be generated around the perimeter of a cell in this field.

Answer 1

`1.32*10^(-11)`

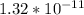
Step-by-step explanation:

Magnetic field
`B=B_(max)sin(wt)`

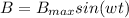
But
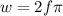`w=2f\pi` and f is given as 69.5Hz
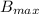`B_(max)` is 0.00100T

Flux through cell,
`\theta=B*Area of cell`

Area of cell=
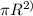`\pi R^{2)` and radius of cell is 6.2µm/2=3.1µm converted to mm it's
`3.1*10^(-6)`

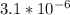
Change in magnetic flux=
`\frac {d\theta}{dt}=\frac {d}{dt}sin wt*\pi R^(2)B_(max)`

`\frac {d\theta}{dt}=w cos wt*\pi R^(2)B_(max)`

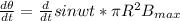
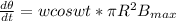
magnitude of induced emf
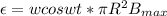`\epsilon= w cos wt*\pi R^(2)B_(max)` where
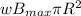`wB_(max)\pi R^(2)` gives maximum value of induced emf

`\epsilon_(max)=wB_(max)\pi R^(2)`

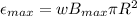
Since
`w=2f\pi=2*69.5* \pi`,
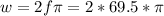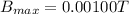`B_(max)=0.00100T` and R=
`3.1*10^(-6)`

`\epsilon_(max)=2*69.5* \pi *0.00100*\pi *(3.1*10^(-6))^(2)`

=
`1.31837*10^(-11)`

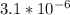
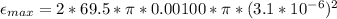
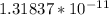
Rounded off to 2 decimal places, max emf=
`1.32*10^(-11)`