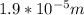Answer:
Step-by-step explanation:
The elongation in bones is calculated from Euler’s equation of
ΔL=
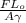 where F is force, A is cross section area, ΔL is elongation of length,
where F is force, A is cross section area, ΔL is elongation of length,
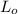 is initial length
is initial length
To find cross sectional area of the bone,
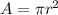 and the radius of the leg is given as 0.018m
and the radius of the leg is given as 0.018m
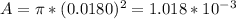
Since the upward force on lower performer is 3 times her weight,
Total force,
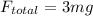 where m is mass of performer provided as 60.0kg
where m is mass of performer provided as 60.0kg
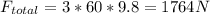
The above force is balanced by two legs hence for each leg,
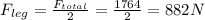
From the formula for elongation initially provided as
ΔL=
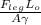
Substituting
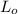 as 0.350m,
as 0.350m,
 as 882N
as 882N

ΔL=
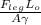
ΔL=
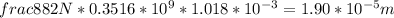
Therefore, elongation is