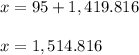Question

The angle of elevation from the top of a 95 foot tall building to a hot air balloon in the sky is 76degree.If the horizontal distance between the building and the hot air balloon is 354feet, find the height of the air balloon.

Answer 1

Answer:
`1,514.816\ feet`

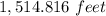
Explanation:

See the image attached, where "x" represents the height in feet of the hot air balloon.

Notice that:

`x=95+BC`

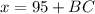
To find the lenght of the side BC of the right triangle, you need to use the following Trigonometric Identity:

`tan\alpha=(opposite)/(adjacent)`

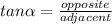
Observe that:

`\alpha =76\°\\opposite=BC\\adjacent=AC=354`

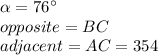
Substituting values and solving for "BC", you get:

`tan(76\°)=(BC)/(354)\\\\(tan(76\°))(354)=BC\\\\BC=1,419.816`

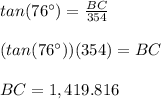
Therefore "x" is:

`x=95+1,419.816\\\\x=1,514.816`