Question

Fatima evaluated the expression when m = -2 and n = 4. Her work is shown below.

What was Fatima’s error?

-She subtracted the exponents incorrectly when simplifying the expression.
-She substituted the wrong values for the variables.
-She applied the exponent -2 to 4(-2) instead of applying the exponent to just -2.
-She found an incorrect value for -8^-2 since the value should be negative.

Answer 1

The answer to your question is: third option is correct.

Explanation:

The third option is correct

4(-2)⁻²(4)⁻³

`(4)/((2)^(2)(4)^(3))}`

Answer 2

Answer: -She applied the exponent -2 to 4(-2) instead of applying the exponent to just -2.

Explanation:

The work of Fatima is the next one:

She starts with the equation:

`(4m^(-3)n^(-2)  )/(m^(-1)n^(2)  )`

Now, she simplifies:

`(4m^(-3)n^(-2)  )/(m^(-1)n^(2)  )` =
`4m^(-3 - (-1)) m^(-2 -1) = 4m^(-2) n^(-3)`

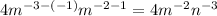
Now she replaces the values of n and m:

`4(-2)^(-2) (4)^(-3)`

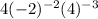
And here she makes her error, she transforms the:

4*(-2)^-2 into (-8)^-2

So the exponent that only was affecting the -2, is now affecting the product 4*(-2)

Then the answer is the third option:

"-She applied the exponent -2 to 4(-2) instead of applying the exponent to just -2."