Question

The College Boards, which are administered each year to many thousands of high school students, are scored so as to yield a mean of 513 and a standard deviation of 130. These scores are close to being normally distributed. What percentage of the scores can be expected to satisfy each of the following conditions? a. Greater than 600 b. Greater than 700 c. Less than 450 d. Between 450 and 600 Bus. 4.71 Monthly sales figures for a particular

Answer 1

a) 25.14% percentage of the scores expected to be greater than 600.

b) 7.49% percentage of the scores expected to be greater than 700.

c) 31.56% of the scores are expected to be less than 450.

d) 43.3% of the scores are expected to be between 450 and 600.

Explanation:

Problems of normally distributed samples can be solved using the z-score formula.

In a set with mean
`\mu` and standard deviation
`\sigma`, the zscore of a measure X is given by

`Z = (X - \mu)/(\sigma)`

After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X. Subtracting 1 by the pvalue, we This p-value is the probability that the value of the measure is greater than X.

For this problem, we have that:

The College Boards, which are administered each year to many thousands of high school students, are scored so as to yield a mean of 513 and a standard deviation of 130, so
`\mu = 513, \sigma = 130`.

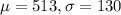What percentage of the scores can be expected to satisfy each of the following conditions?

a) Greater than 600

This is 1 subtracted by the pvalue of Z when
`X = 600`

`Z = (X - \mu)/(\sigma)`

`Z = (600 - 513)/(130)`

`Z = 0.67`

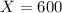
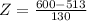
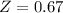
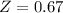`Z = 0.67` has a pvalue of 0.7486.

So there is a 1 - 0.7486 = 0.2514 = 25.14% percentage of the scores expected to be greater than 600.

b) Greater than 700

This is 1 subtracted by the pvalue of Z when
`X = 700`

`Z = (X - \mu)/(\sigma)`

`Z = (700 - 513)/(130)`

`Z = 1.44`

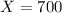
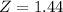
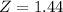`Z = 1.44` has a pvalue of 0.9251.

So there is a 1 - 0.9251 = 0.0749 = 7.49% percentage of the scores expected to be greater than 700.

c. Less than 450

This is the pvalue of Z when
`X = 450`

`Z = (X - \mu)/(\sigma)`

`Z = (450 - 513)/(130)`

`Z = -0.48`

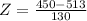
`Z = -0.48` has a pvalue 0.31561.

So, 31.56% of the scores are expected to be less than 450.

d. Between 450 and 600

This is the subtraction of the pvalue of
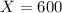`X = 600` by the pvalue of
`X = 450`

So

`P = 0.7486 - 0.31561 = 0.433`

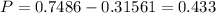
43.3% of the scores are expected to be between 450 and 600.