Question

What is true about △ABC? Select three options

A) AB ⊥ AC
B) The triangle is a right triangle.
C) The triangle is an isosceles triangle.
D) The triangle is an equilateral triangle.
E) BC ∥ AC

Answer 1

Answer:i think its a,b,c

Explanation:

Answer 2

A) AB ⊥ AC

B) The triangle is a right triangle.

C) The triangle is an isosceles triangle

Explanation:

we know that

the formula to calculate the distance between two points is equal to

`d=\sqrt{(y2-y1)^(2)+(x2-x1)^(2)}`

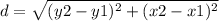
step 1

Find the distance AB

we have

`A(-1,3), B(-5,-1)`

substitute in the formula

`d=\sqrt{(-1-3)^(2)+(-5+1)^(2)}`

`d=\sqrt{(-4)^(2)+(-4)^(2)}`

`d_A_B=√(32)\ units`

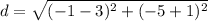
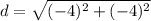
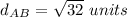
step 2

Find the distance BC

we have

`B(-5,-1),C(3,-1)`

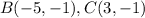
substitute in the formula

`d=\sqrt{(-1+1)^(2)+(3+5)^(2)}`

`d=\sqrt{(0)^(2)+(8)^(2)}`

`d_B_C=8\ units`

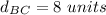
step 3

Find the distance AC

we have

`A(-1,3),C(3,-1)`

substitute in the formula

`d=\sqrt{(-1-3)^(2)+(3+1)^(2)}`

`d=\sqrt{(-4)^(2)+(4)^(2)}`

`d_A_C=√(32)\ units`

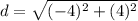
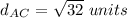
step 4

Compare the length sides of triangle

`d_A_B=√(32)\ units`

`d_B_C=8\ units`

`d_A_C=√(32)\ units`

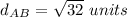
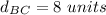
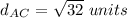
therefore

The triangle ABC is an isosceles triangle, because has two equal sides

The triangle ABC is a right triangle because satisfy the Pythagoras theorem

`BC^2=AB^2+AC^2`

`8^2=(√(32))^2+(√(32))^2`

`64=32+32`

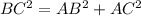
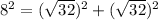
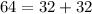
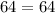`64=64` ----> is true (Is a right triangle)

AB ⊥ AC because in a right triangle the legs are perpendicular