Answer:
A) The time taken by the stone to reach the ground will be 3.257 Seconds
B) The Initial vertical velocity will be zero
C) The horizontal velocity doesn’t change, so it remains the same i.e., 18m/s.
D) The total velocity of the stone just before it hits the water is 36.645 m/s.
A)Explanation :
When the stone is kicked, there are two components act on the stone. One is in the horizontal direction, and the other in downward vertical direction.
Also Initial vertical component of velocity will be zero as the stone is kicked horizontally.
So, the time taken will be given by applying Newton’s Equation of Motion
Or, y=
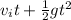
Where y = vertical downward distance
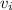 =initial vertical velocity=0 m/s
=initial vertical velocity=0 m/s
t = time taken to reach the ground
Substituting the values in the above equation, we find
-52 = 0 +

or,
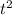 =
=
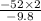
or, t =
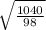 = 3.257 s
= 3.257 s
B) Explanation
The Initial vertical velocity will be zero as the stone is kicked horizontally
C)Explanation
The horizontal velocity component is not under the influence of gravity, so it remains the same, and moves constant velocity horizontally.
D) Explanation
We will firstly find the final vertical velocity using one of the equations of motion
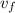 =
=
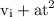
Substituting the values in the above equation, we find
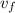 =
=
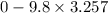 =-31.92 m/s
=-31.92 m/s
Now, Total Velocity (v) is given by
v =
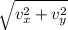
where,
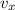 = Horizontal velocity
= Horizontal velocity
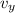 = Vertical velocity
= Vertical velocity
Substituting the values in the above equation, we find
v =
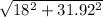 = 36.645 m/s
= 36.645 m/s