Question

A wet bar of soap slides down a ramp 9.2 m long inclined at 8.0∘ .

How long does it take to reach the bottom? Assume μk = 0.056.
Express your answer to two significant figures and include the appropriate units.

Answer 1

The time taken by the bar to reach the bottom t=4.886s

Given:

Displacement of the bar S=9.2m

Angle of inclination
`\theta=8.0^(\circ)`

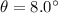
Coefficient of friction factor
`\mu k=0.056`

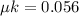
To find:

How long it takes to reach the bottom ‘t’

Step by Step Explanation:

Solution:

We know that the formula for weight of the soap bar is given as

`F_(g)=m g \sin \theta`

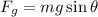
The frictional force acting on this soap bar is determined by

`F_(f)=\mu m g \cos \theta`

To determine the constant acceleration of the bar, we derive as

`F=m a`

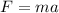
Here
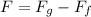`F=F_(g)-F_(f)` and thus

`F_(g)-F_(f)=m a`
`m g \sin \theta-\mu m g \cos \theta=m a`

`a=g \sin \theta-\mu g \cos \theta`

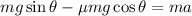
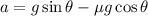
Where
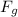`F_(g)`=Force imparted due to weight

`F_(f)`=Frictional Force

m=Mass of the bar

g=Acceleration due to gravity

a=Acceleration of the bar

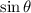`\sin \theta` and
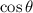`\cos \theta` are the angles involved in the system

If the bar starts from the rest

Equations of motion involved in calculating the displacement of the bar is given as

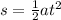`s=(1)/(2) a t^(2)`, From this

`a t^(2)=2 s`

`t^(2)=(2 s)/(a)`

`t=\sqrt{(2 s)/(a)}`

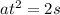
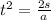
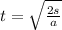
Where s= displacement or length moved by the bar

a=Acceleration of the bar

t=Time taken to reach bottom

Substitute all the known values in the above equation we get

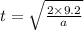`t=\sqrt{(2 * 9.2)/(a)}` and we know that

`a=g \sin \theta-\mu g \cos \theta`

`=9.8 * \sin 8.0-0.056 * 9.8 * \cos 8.0`

`=1.364-0.543`

`a=0.821`

`t=\sqrt{(2 * 9.2)/(0.821)}`

`t=\sqrt{(19.6)/(0.821)}`

`t=√(23.87332)`

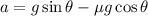
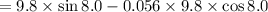
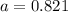
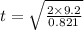
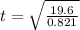
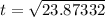
t=4.886s

Result:

Thus the time taken by the bar to reach the bottom is t=4.886s