Answer:
2.47 m
Step-by-step explanation:
Let's calculate first the time it takes for the ball to cover the horizontal distance that separates the starting point from the crossbar of d = 52 m.
The horizontal velocity of the ball is constant:

and the time taken to cover the horizontal distance d is
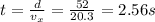
So this is the time the ball takes to reach the horizontal position of the crossbar.
The vertical position of the ball at time t is given by
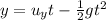
where
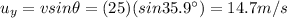 is the initial vertical velocity
is the initial vertical velocity
g = 9.8 m/s^2 is the acceleration of gravity
And substituting t = 2.56 s, we find the vertical position of the ball when it is above the crossbar:
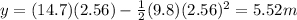
The height of the crossbar is h = 3.05 m, so the ball passes

above the crossbar.