Question

Mr Magee wrote the expression shown Below on the board if the expression has a value of 8 which of the following is the value of n​

Answer 1

Explanation:

512^(1/n) = 8

(2⁹)^(1/n) = 2³

2^(9/n) = 2³

=> 9/n = 3, n = 3. (D)

P.S.

Law of Indices: (a^m)^n = a^(mn)

Answer 2

The expression has a value of 8, then n is 3.

The expression
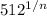`512^{1/n` can be rewritten as the nth root of 512. In other words, you are looking for the value of n such that the nth root of 512 is equal to 8.

Mathematically, this can be represented as:

`\sqrt[n]{512 =8}`

To find the value of n, you need to think about what power or root results in 8 when applied to 512. In this case, n would be 3 because 8^3 =512.

So, if the expression has a value of 8, then n is 3.