Answer:
The next three terms of the geometric sequence are 875, 175, 35, 7 is 1.4, 2.8, and 0.56.
Solution:
Given Geometric sequence is 875, 175, 35, 7.
We need to find the next three sequence.
A geometric sequence is one where any value in the sequence can be determined using the formula:

Where
n is the nth term in the sequence,
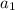 is the first term (in this case, 875) and "r" is the rate of change between them.
is the first term (in this case, 875) and "r" is the rate of change between them.
To find r, you simply divide the second term by the first:

Inserting
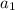 = 875 and r=0.2 into the formula above, you have the equation for the sequence:
= 875 and r=0.2 into the formula above, you have the equation for the sequence:
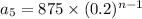
To find 5th sequence:

To Find 6th Sequence:
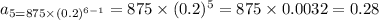
To Find 7th Sequence:
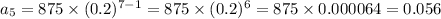
Hence the next three terms of the geometric sequence are 875, 175, 35, 7, 1.4, 0.28, and 0.056.