Answer:
a.No
b.No
c.Yes
Explanation:
a.We are given that a fraction number
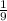
We have to find that is exact decimal or not.
Calculator display for
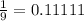
It is not an exact decimal equivalent.
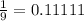
A fraction is an exact decimal when the denominator can be expressed as
 .
.
9 cannot be expressed as

Hence,
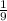 is not an exact decimal equivalent.
is not an exact decimal equivalent.
b.
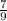
Calculator display for

It is not an exact decimal equivalent because the denominator of given fraction is 9 which can not be expressed as
 .
.
Hence, it is not an exact decimal equivalent.
c.
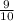
Calculator display for
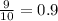
10=
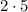
It is an exact decimal equivalent because the denominator 10 can be expressed as
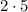 .
.
Hence, the given decimal is an exact decimal equivalent.