Answer:
See graph of the two conics in the attached picture
Explanation:
We create two vertices for the ellipse on the y-axis located at the points (0,2) and (0, -2) (marked with orange dots in the picture) and then create the equation for the ellipse such that its focus coincide with the place for the trees as requested. Recalling the formula for the position of the focus (c) for a horizontal ellipse of the form:
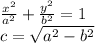
Since our
 value is 2 (choice of two vertices above), we have:
value is 2 (choice of two vertices above), we have:
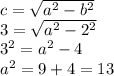
So now we can complete the equation of our ellipse with the trees at (-3,0) and (3,0):
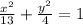
Now we create the horizontal hyperbola of general form:
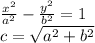
We request the same values for "c" and for "b" as we did with the ellipse:
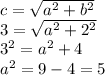
So now we can write the equation for this horizontal hyperbola:
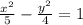
Both conics are plotted in the attached image, and their respective vertices that correspond to
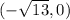 and
and
 for the ellipse, and
for the ellipse, and
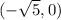 and
and
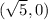 for the hyperbola, are indicated with blue dots and orange dots respectively.
for the hyperbola, are indicated with blue dots and orange dots respectively.
The location of the trees (focus) is indicated with red dots.