ANSWER:
The value of (f + g)(6) = 28
SOLUTION:
Given that f(x) = -x-2 and g(x) =

We need to find the value of (f+g)(6)
(f + g)(x) is an arithmetic combination of f(x) and g(x)
As, the operator between f and g is addition operator, the value of arithmetic combination becomes
(f + g)(x) = f(x) + g(x)
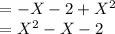
Now, put x = 6 in (f + g)(x)
(f + g)(6) =
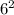 – 6 – 2
– 6 – 2
= 36 – 6 – 2
= 36 – 8 = 28
Hence, the value of (f + g)(6) = 28